- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2004
- X-ray Absorption and Magnetic Scattering
- f-State Occupancy at the GAMMA-ALPHA Phase Transition of Ce-Th and Ce-Sc Alloys
f-State Occupancy at the GAMMA-ALPHA Phase Transition of Ce-Th and Ce-Sc Alloys
The Ce ![]() -
-![]() transition is one of the most intriguing phenomena in condensed matter physics. It embraces a variety of complex mechanisms, which generate anomalous behaviour both on a structural level (lattice parameter), and, more fundamentally, in the magnetic (magnetisation) and electronic properties (specific heat, etc.). There is a solid consensus even beyond the widely discussed Mott transition and the Kondo volume collapse paradigms that hybridisation between 4f and valence electrons plays a key role in the phase transition. In particular, the large Ce
transition is one of the most intriguing phenomena in condensed matter physics. It embraces a variety of complex mechanisms, which generate anomalous behaviour both on a structural level (lattice parameter), and, more fundamentally, in the magnetic (magnetisation) and electronic properties (specific heat, etc.). There is a solid consensus even beyond the widely discussed Mott transition and the Kondo volume collapse paradigms that hybridisation between 4f and valence electrons plays a key role in the phase transition. In particular, the large Ce ![]() -
-![]() volume collapse (
volume collapse (![]() V/V0 ~14%) is supposedly driven by a change in interactions involving f orbitals.
V/V0 ~14%) is supposedly driven by a change in interactions involving f orbitals.
Hybridisation may be defined formally in terms of configuration interaction. Then, the ground state can be written as a linear combination of unoccupied, singly and doubly occupied f states. Obtaining an exact description of the cerium ground state implies a precise "counting" of the 4f electrons. X-ray absorption spectroscopy (XAS) and X-ray photoemission (XPS) experiments have been widely used to that purpose over the past few years. Nevertheless, both fail to provide a precise electron count for cerium: the f2 contribution is hidden in XAS due to lifetime broadening, and surface effects in XPS lead to an artificial reinforcement of the Ce-g character.
Resonant X-ray inelastic scattering (RIXS) turns out to be a powerful alternative, thanks to the resonant enhancement and sub-lifetime resolving power. We have applied 2p3d-RIXS in Ce-Th, and Ce-Sc alloys, where the ![]() -
-![]() transition is driven by temperature using the doping element for chemical pressure [1]. The experiment was carried out at ID12, using our UHV-compatible RIXS spectrometer. The Ce-La emission line was measured on resonance in the vicinity of the Ce L3-edge. Figure 130 illustrates the 2p3d-RIXS spectra obtained in Ce0.9Th0.1 at ambient temperature.
transition is driven by temperature using the doping element for chemical pressure [1]. The experiment was carried out at ID12, using our UHV-compatible RIXS spectrometer. The Ce-La emission line was measured on resonance in the vicinity of the Ce L3-edge. Figure 130 illustrates the 2p3d-RIXS spectra obtained in Ce0.9Th0.1 at ambient temperature.
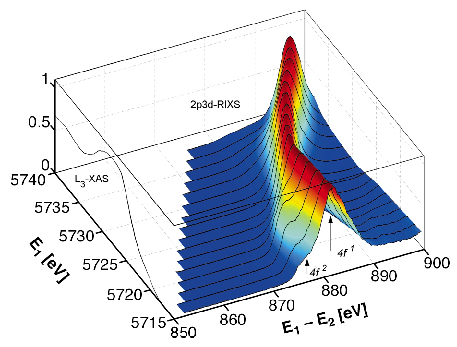 |
|
Fig. 130: 2p3d-RIXS spectra in Ce0.9Th0.1 represented in the incident (E1) vs transfer energy (E1-E2) plane. |
The spectra are arranged in a surface plot, laid out in the incident energy (E1) vs transfer energy (E1-E2) plane. At low E1, the RIXS spectra show two distinct features, attributed to the 4f1vn+1 and 4f2vn final state configurations (i.e. with an extra valence electron in the valence band v, and in presence of a 3d core hole). As the incident energy increases, a clear resonance of the 4f2 features occurs, which permits an accurate estimate of the f1 and f2 spectral weights across the transition.
The temperature dependence of the f1/f2 intensity ratio is represented in Figure 131 for the three alloys. All the samples show a sharp decrease at the transition temperature and hysteresis reminiscent of a first-order transition. The hysteresis loops present striking similarities with bulk magnetisation measurements plotted below. Obviously the magnetisation is a ground-state property whereas RIXS involves excited final states. Even so, the resemblance between the two sets of data is not coincidental and underlines the intimate relationship between the RIXS data and the anomalous behaviour at the transition. This assumption is confirmed when comparing the f1/f2 intensity ratio to first-principle calculations of the cerium ground-state properties obtained by LDA+DMFT [2]. To retrieve the correct intensity ratio, underestimated in the raw data due to tail excitation effects, we have simulated the RIXS spectra within a phenomenological approach. Both simulated and calculated results show a fair agreement when rescaled to the total volume change, especially for the Th-doped samples. The departure from the calculated value in Ce-Sc may be ascribed to interactions with Sc-3d electrons.
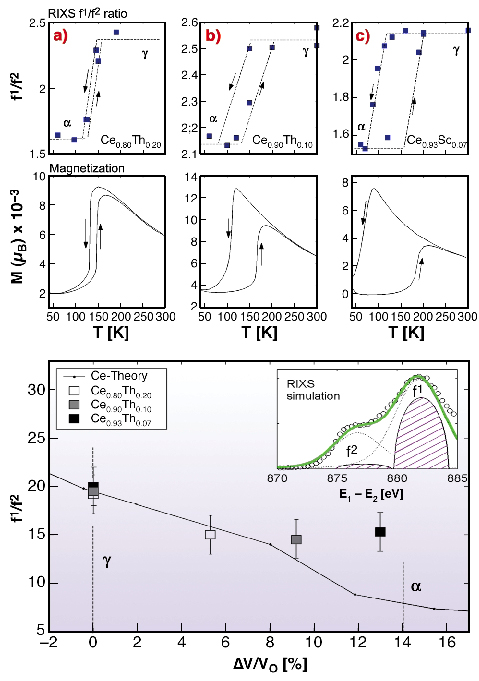 |
|
Fig. 131 f1/f2 ratio obtained by RIXS (upper) and bulk magnetisation measurements (middle). Corrected ratio derived from a RIXS simulation compared to ab-initio calculations from Ref.[2] (lower). |
These results demonstrate that the f2 contribution cannot be neglected in the description of the Ce ground state, and underlies the importance of the f electron screening. More generally, such an experiment should further establish RIXS as a major spectroscopic method to study mixed-valent systems.
References
[1] K.A. Gschneider, R.O. Elliott and R.R. McDonald, J. Phys. Chem. Solids 23, 555 (1962).
[2] A.K. McMahan, K. Held, and R.T. Scalettar, Phys. Rev. B 67, 075108 (2003).
Principal Publications and Authors
J.-P. Rueff (a), C. F. Hague (a), J.-M. Mariot (a), L. Journel (a), R. Delaunay (a), J.-P. Kappler (b), G. Schmerber (b), A. Derory (b), N. Jaouen (c), and G. Krill (d), Phys. Rev. Lett. 93, 067402 (2004).
(a) Laboratoire de Chimie Physique - Matière et Rayonnement, CNRS - Université Paris 6 (France)
(b) Institut de Physique et Chimie des Matériaux, CNRS Université Strasbourg (France)
(c) ESRF
(d) Laboratoire de Physique des Solides, CNRS Université Paris Sud, Orsay (France)



