- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2016
- Complex systems and biomedical sciences
- Correlation between structural changes and atomic motion during physical ageing in metallic glasses
Correlation between structural changes and atomic motion during physical ageing in metallic glasses
A link between the microscopic structural mechanisms and atomic motion in metallic glasses has been discovered by a combination of dynamical and structural synchrotron techniques, providing a unique and broader view of the atomic-level complexity of these unique materials.
Metallic glasses display outstanding thermal, mechanical and chemical properties that make them forefront materials for technological applications. However, their widespread use is limited by their lack of stability over time due to physical ageing, i.e. the spontaneous temporal evolution of any functional physical property due to relaxation processes intrinsic to the glassy state [1,2].
We have provided an overview and an atomistic understanding of the complexity of ageing in a metallic glass by connecting microscopic structural re-arrangements and atomic motion for the first time. We found that the atomic scale is dominated by the interplay between stress-releasing rearrangements leading to density inhomogeneity annihilation and fast dynamical regimes of ageing and medium range ordering processes, not affecting density, related to a more localised atomic motion. The evolution between these regimes is probably associated with a ductile-to-brittle transition.
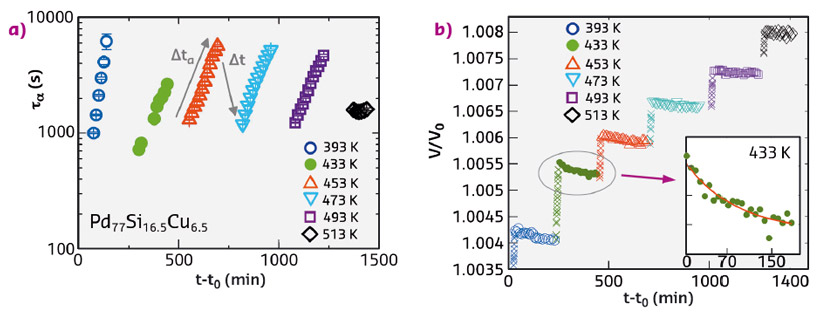
To clarify the unique atomic motion in metallic glasses [3], we have investigated the microscopic structural and dynamical changes occurring in a rapidly quenched Pd77Si16.5Cu6.5 metallic glass (glass transition temperature Tg = 625 K), during successive annealing below Tg, using X-ray photon correlation spectroscopy (XPCS) at beamline ID10. Figure 104a shows structural relaxation times ταas a function of the time elapsed from the beginning of the heating protocol. This parameter represents the characteristic time for the dynamics at the probed length-scale. For T < 513 K, τα increases exponentially during the isotherm signalling a non-stationary dynamics, while it abruptly decreases during T changes due to the increasing thermal motion. The exponential increase corresponds to the fast ageing regime observed also in other hyper-quenched metallic glasses [3], which can be described by the relation τα(ta,T)~exp(ta/τ*), with an almost constant growth rate parameter τ*~6000 s. At T = 513 K, the ageing abruptly stops even if the system is well below Tg: the glass enters a stationary regime where τα remains constant at least on the probed experimental timescale.
 |
|
Fig. 104: a) Time and temperature evolution of the structural relaxation time measured with XPCS as a function of t-t0, where t0 is the time corresponding to the beginning of the heating protocol. b) Relative volume change, reported as a function of t-t0. Each temperature ramp (x) is followed by an isotherm Inset: zoom of the curve at 433 K reported as a function of time from the beginning of the isotherm, together with the best fit to an exponential law. |
Figure 104b presents the corresponding volume evolution as tracked by the position change of the first sharp diffraction peak (FSDP) measured by high-resolution high-energy X-ray diffraction (XRD) at beamline ID15B. During isotherms, the volume decreases slightly, most likely corresponding to the annihilation of residual density inhomogeneity. For increasing temperatures, the total isothermal densification decreases. Simultaneously to the onset of the stationary regime in dynamics, at T = 513 K, the volume reduction stops, corresponding to full structural defect annihilation. This suggests that structural defect annihilation is the process responsible for the fast ageing.
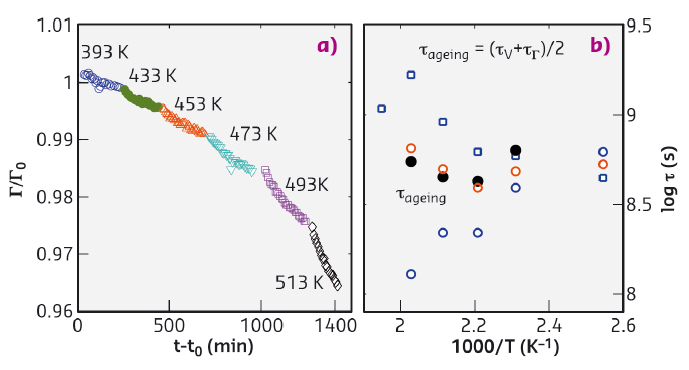
As shown in Figure 105a, a continuous narrowing of the FSDP takes place from the ageing itself, which continues even when there is no further densification. This narrowing can be taken as an indicator of an increasing medium range order, for r ≥ 6 Å [4].
 |
|
Fig. 105: a) Temporal evolution of the relative change of the width of the FSDP. b) Characteristic time for ageing as obtained from the volume relaxation (blue squares), the narrowing of the FSDP (blue circles) and from XPCS data (black dots). The average of τV and τΓ is also shown (red circles), called τageing. |
Both the volume and the FSDP width can be described by an exponential law with characteristic times τV and τΓ, respectively. While the volume and FSDP width have a different T dependence from the dynamical time for ageing τ*, the agreement between τ* and their average is striking (Figure 105b), suggesting that the fast dynamical ageing is due to both processes: structural defect annihilation and medium range ordering to the extent that they affect the density.
Once the structural defects are completely annihilated, the density is frozen. and thus XPCS does not see further ageing and the stationary regime is entered where dynamical measurements indicate that other stresses still exist, most likely from frustration in the repetition and ordering of atomic clusters.
These results provide a direct connection between dynamical and structural microscopic evolution in metallic glasses which is fundamental for the development of a microscopic theory of ageing and ultimately designing new amorphous materials with improved stability.
Principal publication and authors
Unveiling the structural arrangements responsible for the atomic dynamics in metallic glasses during physical aging, V.M. Giordano (a) and B. Ruta (b), Nature Communications 7, 10344 (2016); doi: 10.1038/NCOMMS10344.
(a) Institute of Light and Matter, UMR5306 Université Lyon 1-CNRS, Université de Lyon, Villeurbanne, (France)
(b) ESRF
References
[1] Y. Zhang et al., Nat. Mat. 5, 857 (2006).
[2] W.H. Wang, Prog. Mater. Sci. 57, 487 (2012).
[3] B. Ruta et al., Phys. Rev. Lett. 109, 165701 (2012).
[4] D. Ma et al., Nat. Mater. 8, 30 (2009).



