- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2012
- Structure of materials
- Crystallisation during bending of a metallic glass
Crystallisation during bending of a metallic glass
Atoms in metallic glasses are arranged in no particular long-range order and instead form a rather random packing of compact clusters [1], without the availability of slip systems and dislocation glide for plastic deformation as in crystalline materials which have long-range lattice order. Their disorder endows metallic glasses with high resilience up to elastic strains of 2% or more before undergoing heterogeneous plastic deformation via the formation of shear bands.
Shear bands are thin (20-50 nm), nearly planar zones of intense plastic deformation where dissipation of mechanical work generates free volume [2] and results in local heating estimated to be anywhere from negligible to reaching above the melting temperature Tf [3]. Crystallite formation after plastic deformation has been detected by transmission electron microscopy (TEM) but only after specimen thinning followed by electron-beam focusing on regions of shear bands, and many experimental artifacts must be excluded.
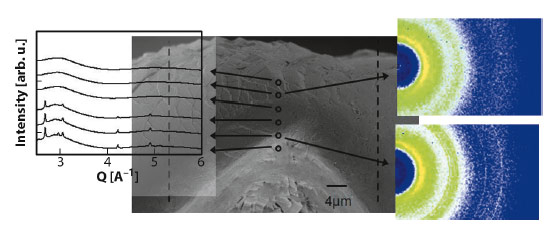
We performed non-destructive diffraction on a bent 35 µm thick glassy Pd40Cu30Ni10P20 ribbon in transmission geometry using a monochromatic beam at beamline ID11. The incident beam was focused to 2 µm in diameter for microprofiling across the bent specimen width every few micrometres along the thickness. Figure 119 shows the resulting X-ray microscopy images together with a scanning electron microscope (SEM) image of the bend in the ribbon. Crystalline Bragg peaks are seen to appear on the compressive fibre side of the bend but not on the tension side.
 |
|
Fig. 119: X-ray microscopy images during microprofiling along the thickness through the width of bent glassy Pd40Cu30Ni10P20 ribbon. |
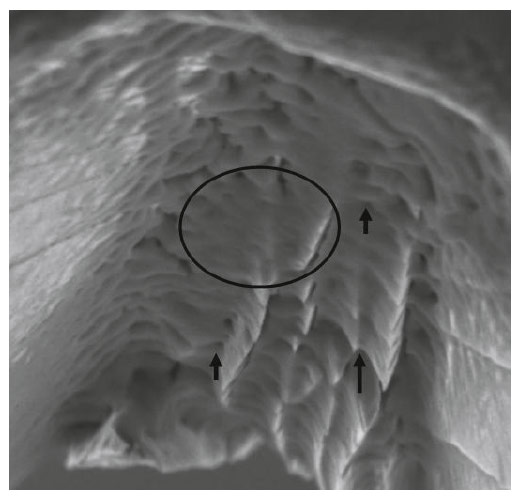
Figure 120 is a close-up SEM image of the inner (compressive) side of the bend in the ribbon showing shear steps with viscous shapes and meniscus formation [3].
 |
|
Fig. 120: SEM image of the inner surface of bent glassy Pd40Cu30Ni10P20 ribbon showing shear steps with viscous shapes and meniscus formation. |
What remained to be explained was why crystallisation occurs on the compression side and not on the tension side of the bend. Homogeneous nucleation frequency per volume per time Ivhom(T) in the so-called steady-state regime is formulated as:
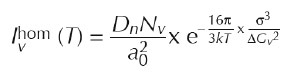 |
|
Equation (1) |
where Dn, Nv and a0 are respectively an atomic diffusivity, number of atoms per unit volume and an average inter-atomic distance. σ is the crystal-liquid interfacial energy, ∆Gv the driving free energy for crystallisation per unit volume, T the temperature and k the Boltzmann constant. Eq. (1) can be modified for crystallisation under external hydrostatic compressive pressure P as:
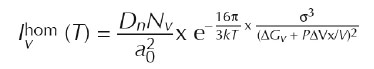 |
|
Equation (2) |
where ∆Vx/V is the usually negative reduced volume-change on crystallisation and the term P∆Vx/V comes as a modification of ∆Gv due to a pressure-induced increase in the melting temperature Tm (or an increase in undercooling) as formulated in the Clapeyron-Clausius formula [4].
A glassy ribbon reaches plastic yield resulting in the formation of shear bands under an applied stress τ ≈ τy. For the PdCuNiP family of metallic glasses τy ≥ 1.5 GPa. When temperature T reaches that of crystallisation Tx ≈ 700 K as determined by calorimetry, we can write:
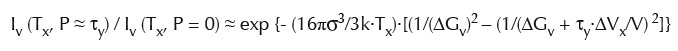 |
|
Equation (3) |
Using the materials parameters listed in the principal publication, we obtain Iv (Tx, τy) / Iv (Tx) ≈ 1055 as compared to Ivhom (700K) ≈ 10-5 /m3·s without any external stress. Thus, in the compression side of the bend, the value could reach 1050 /m3·s.
The above numbers show high nucleation frequencies because a large volume change ∆Vx/V ≈ –2% on crystallisation at Tx ≈ 700 K as compared with a much smaller volume change of below 1% at room temperature. The larger volume change ∆Vx/V is due to the large thermal expansion coefficient of the liquid state and an extended supercooled liquid region ∆T stretching about 100 K between Tg and Tx.
The analysis suggests that a wide supercooled liquid region is required for stress-crystallisation of a metallic glass deformed in compression. This confirms previous suggestions that shear band crystallisation contributes to a more extended strain which causes the fracture of metallic glasses under compressive load by shear delocalisation [5], a mechanism that does not intervene under tension.
Principal publication and authors
A.R. Yavari (a,b), K. Georgarakis (a,b), J. Antonowicz (c), M. Stoica (d), N. Nishiyama (b), G. Vaughan (e), M. Chen (b) and M. Pons (a), Phys. Rev. Lett. 109, 085501 (2012).
(a) Euronano SIMaP-CNRS, Institut Polytechnique de Grenoble INPG (France)
(b) WPI AIMR Tohoku University (Japan)
(c) Faculty of Physics, Warsaw University of Technology (Poland)
(d) Institute for Complex Materials, IFW Dresden (Germany) (e) ESRF
References
[1] A.R. Yavari, Nature 439, 405 (2006).
[2] A.R. Yavari, A. Le Moulec, A. Inoue, N. Nishiyama, N. Lupu, E. Matsubara, W.J. Botta, G. Vaughan, M. Di Michiel and Å. Kvick, Acta Materialia 53, 1611 (2005).
[3] K. Georgarakis, M. Aljerf, Y. Li, A. LeMoulec, F. Charlot, A.R. Yavari, K. Chornokhvostenko, E. Tabachnikova, G.A. Evangelakis, D.B. Miracle, A.L Greer and T. Zhang, Appl. Phys. Lett. 93, 031907 (2008).
[4] L.D. Landau and E.M. Lifshitz, “Statistical Physics”, Addison-Wesley Publishing, p. 261 (1974).
[5] K. Hajlaoui, A.R. Yavari, B. Doisneau, W.J. Botta, W. Zhang, G. Vaughan, A. Kvick, A. Inoue and A.L. Greer, Mater. Sci. Eng. A 105, 449–451 (2007).



