- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2004
- Materials Science
- In situ Measurement of Dynamic Precipitation in an Al-Zn-Mg-Cu Aluminium Alloy
In situ Measurement of Dynamic Precipitation in an Al-Zn-Mg-Cu Aluminium Alloy
Aluminium alloys are frequently alloyed to give rise to precipitation hardening, increasing the strength of these light alloys to more than 600 MPa. It is known that the presence of dislocations modifies the precipitation reaction profoundly in such materials, promoting heterogeneous precipitation of a stable phase, whereas the material free of crystalline defects experiences the formation of metastable precursors [1] such as the Guinier Preston (GP) zones in Al-Cu alloys.
When dislocations move into a supersaturated solid solution (when it is being plastically deformed), a significant acceleration of the precipitation kinetics can be observed. Although this phenomenon is well known at high temperatures (above 300°C) [2], where diffusion is very fast, it was not known whether this happens at ambient temperatures. In fact, the precipitates that form during static ageing (i.e. without plastic deformation) at room temperature are extremely small, and can only be observed using small-angle scattering techniques.
We have studied dynamic precipitation at ambient temperature in an Al-Zn-Mg-Cu alloy (alloy 7010, a type used for airplane wings), quenched from 475°C to room temperature. Precipitate size and volume fraction were determined by small-angle X-ray scattering (SAXS) performed on the D2AM (BM02) beamline at an energy of ~8 keV. Plastic deformation has been performed in situ on the 100 µm thick samples, controlling the amount of deformation by monitoring the sample transmission during the test. X-rays are collected by a CCD camera and a radial average is performed to obtain normalised scattering intensity profiles.
After quenching, the material gradually evolves forming fine scale precipitates (GP zones containing all three alloying elements) ; the nucleation radius is about 0.5 nm, and the volume fraction evolves in an hour to more than 2% (Figure 34).
 |
|
Fig. 34: Evolution of the precipitate size and volume fraction at ambient temperature following a quench from 475 °C (SAXS measurements). |
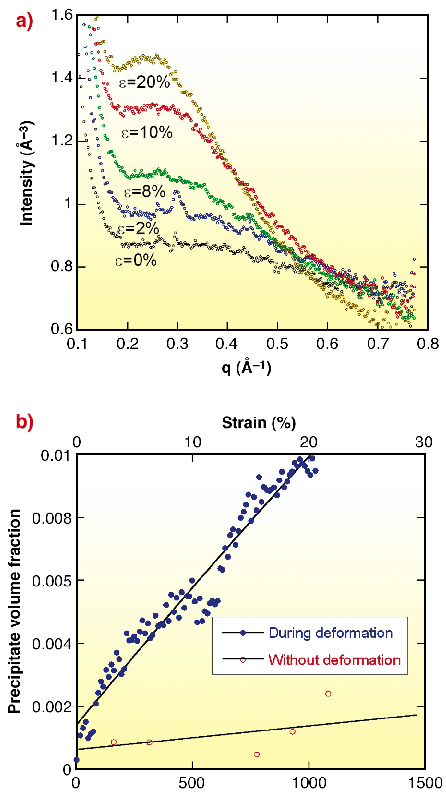
During straining, a fast increase in scattered intensity is observed (Figure 35), which is evidence for the occurrence of precipitation. However, this precipitation has to be compared to that which occurs if the sample is not deformed on the same time scale. Figure 35b shows that the dynamic precipitation process is almost an order of magnitude faster than static ageing. Moreover, we have characterised a strong negative strain rate sensitivity of this phenomenon: above a strain rate of ~ 5. 10-3 s-1, it becomes almost negligible. This strain rate sensitivity is coherent with a mechanism similar to dynamic strain ageing [3]: precipitates form during the waiting time of dislocations at obstacles, which itself depends on the strain rate.
 |
|
Fig. 35: (a) SAXS profiles as a function of plastic strain show evidence for the apparition of small precipitates; (b) the precipitate volume fraction is plotted both as a function of strain and time. The latter presentation enables the comparison with static precipitation (data from Figure 34), showing the large increase in precipitate kinetics associated with the dynamic precipitation. |
The direct evidence of dynamic precipitation is an important step in understanding some remarkable properties of supersaturated solid solutions, and notably the very high strain hardening rate (slope of the stress-strain curve) observed [4], which is twice the value predicted by the accepted internal variable theory. Precipitates formed during straining participate subsequently to the flow stress. The negative strain rate sensitivity of dynamic precipitation also explains the corresponding negative strain rate sensitivity of the strain hardening rate.
References
[1] F.C. Larché, in Dislocations in Solids, North-Holland Publ. Co, Amsterdam, Netherlands, 4, 135 (1979).
[2] E. Evangelista, H.J. Mcqueen, and E. Cerri, in Proc. of the 13th Riso Int. Symp. on Mat. Sc., Risø National Laboratory, Roskilde, Denmark, 265 (1992).
[3] L.P. Kubin, and Y. Estrin, Y., Acta Metall. Mater. 38 (5) 697 (1990).
[4] W.J. Poole, and H.R. Shercliff, Mat. Science Forum, 217-222, 1287(1996).
Principal Publication and Authors
A. Deschamps (a), F. Bley (a), F. Livet (a),D. Fabregue (a), L. David (b), Phil. Mag. A 83 (6), 677-692 (2003).
(a) LTPCM / INPG (France)
(b) GEMPPM, INSA Lyon (France)



