- Home
- Users & Science
- Scientific Documentation
- ESRF Highlights
- ESRF Highlights 2007
- Surface and Interface Science
- Probing a surface reconstruction with anomalous X-ray diffraction
Probing a surface reconstruction with anomalous X-ray diffraction
Surface X-ray diffraction (SXRD) is a powerful technique for the determination of surface structures. Whereas most traditional electron or ion based surface science techniques are limited to the vacuum environment, SXRD is able to determine structural parameters of surfaces under a gas pressure of several bar, or from a surface immersed in a liquid because of the negligible attenuation of hard X-rays. One drawback with SXRD is the often limited data set that can be collected during an experiment when compared to a more traditional technique such as low energy electron diffraction (LEED). This limits the uniqueness of the resulting structure determined on trial and error basis. This limitation can be reduced by the use of a two-dimensional detector, allowing a greater number of data points to be extracted in a shorter time span. Furthermore, SXRD can be combined with other experimental techniques, or with theoretical ab initio calculations based on DFT (density functional theory). The data set can also be extended by using anomalous diffraction [1].
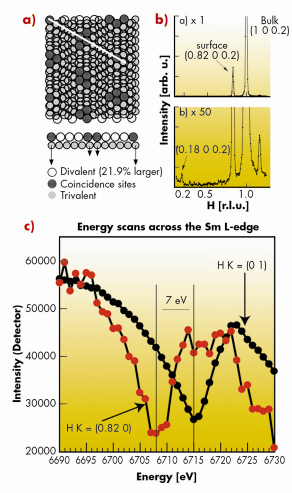
In the present study we have investigated the surface reconstruction of Sm [2]. In this system, the bulk Sm atoms are trivalent (4f5(6s6p5d)3) and the surface atoms are divalent (4f6(6s6p5d)2). This valence transition, which is unique among the pure elements, leads to some very unusual surface properties, the most prominent being an expansion of the divalent surface Sm atoms by 22%, leading to a coincidence lattice. We have previously studied the resulting surface reconstruction in great detail, as summarised in Figure 97a. The correct identification of the structure was supported by ab-initio theoretical calculations.
 |
|
Fig. 97: (a) Top and side views of the surface reconstruction of Sm(0001). (b) In-plane H-scan (K=0) clearly showing the incommensurate surface layer. (c) Photon energy scans across the Sm L-edge at (H K L) = (0.82 0 0.2) (red-dotted line) and at (H K L) = (0 1 0.2) (black-dotted line) corresponding to diffraction from the surface and the bulk, respectively. The shift between the dips at resonance corresponds to the core level binding energy shift between divalent and trivalent Sm atoms as found by xps [3]. |
In this structure, the different in-plane nearest-neighbour distance in the top-most layer with respect to the bulk layers can easily be detected using SXRD, Figure 97b. We have an extremely clear signal for differentiating between the single divalent surface layer and the deeper trivalent bulk layers. The window free design of the refurbished ID03 beamline permitted us to reach the quadrupole (2p – 4f) resonance at 6.716 keV for trivalent Sm and perform surface resonant scattering from this single, well-defined surface layer. In a first experiment we detected the diffracted intensities from the bulk signal at H = 0 and K = 1 (L = 0.2), and scanned across the L-edge, as shown by the black dotted line in Figure 97c. A clear dip is seen at a photon energy of 6715 eV. If instead we detect the diffracted photons from the surface signal at H = 0.82 and K = 0, a clear shift of the dip to 6708 eV can be observed in the red dotted line in Figure 97c. Thus we find a shift between the bulk and the surface anomalous diffraction of approximately 7 eV. This value is very close to the shift in the 3d level between divalent and trivalent Sm atoms [3], directly showing that the surface signal stems from divalent Sm atoms while the bulk signal is from trivalent Sm atoms. The experiment thus confirms previous surface models for the valence induced surface reconstruction of Sm. It also demonstrates the ability of the ID03 beamline to scan the photon energy in a controlled manner, and the ability to reach the lower-lying (2p-4f) resonances in the important rare earth materials.
Anomalous SXRD can be used at ID03 in a simple way to gain additional, and sometimes essential chemical information on an unknown surface structure. A detailed structural evaluation based on surface anomalous diffraction must include the photon energy dependence of the transmission function and the form factors involved. In addition, the sensitivity to anomalous scattering of the topmost reconstructed surface layer may be used to perform resonant magnetic diffraction measurements, allowing an accurate isolation and quantification of the magnetisation of the topmost atomic plane.
Reference
[1] B.E. Warren, X-ray Diffraction, Dover Publications Inc. (1990).
[2] E. Lundgren, J.N. Andersen, R. Nyholm, X. Torrelles, J. Rius, A. Delin, A. Grechnev, O. Eriksson, C. Konvicka, M. Schmid, P. Varga, Phys. Rev. Lett. 88 136102 (2002).
[3] Å. Fäldt and H. Myers, Phys. Rev. B 33, 1424 (1986).
Principal publication and authors
E. Lundgren (a), R. Westerström (a), J.N. Andersen (a), X. Torrelles (b), C. Quiros (c), S. Ferrer (d), I. Popa (e), D. Wermeille (e), R. Felici (e), to be published.
(a) Department of Synchrotron Radiation Research, Lund University (Sweden)
(b) Institut the Ciencia de Materials de Barcelona (Spain)
(c) Departamento de Física, Universidad de Oviedo (Spain)
(d) ALBA, Barcelona (Spain)
(e) ESRF



